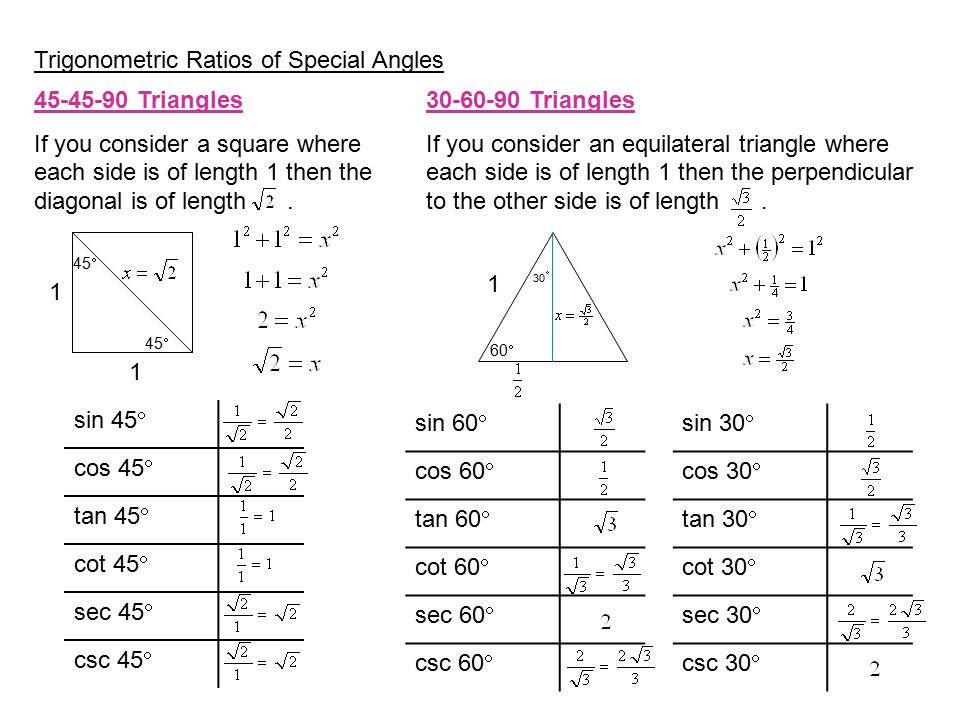
Trigonometric ratios are Sine, Cosine, Tangent, Cotangent, Secant and Cosecant The standard angles for these trigonometric ratios are 0 °, 30°, 45°, 60° and 90° These angles can also be represented in the form of radians such as 0, π/6, π/4, π/3, and π/2THE 30°60°90° TRIANGLE THERE ARE TWO special triangles in trigonometry One is the 30°60°90° triangle The other is the isosceles right triangle They are special because, with simple geometry, we can know the ratios of their sides Theorem In a 30°60°90° triangle the sides are in the ratio 1 2 We will prove that below2 The triangle Begin with an isosceles right triangle (construct a segment, rotate it 90 degrees, connect the two remaining vertices
Special Right Triangles Proof Part 1 Video Khan Academy
Trigonometric ratios 30-60-90 triangle
Trigonometric ratios 30-60-90 triangle-A proof that sin(30) = 1/2, cos(30) = √3/2, sin(60) √3/2, cos(60) = 1/2 is as follows Consider an equilateral triangle, all angles are 60º Drop a bisector from one of the 60º angles, it will also be a perpendicular bisector to its opposite sideAnswer (1 of 5) The length of the two sides of a 30°_60°_90° right triangle are 4 inches and 4√3 inches What is the length of its hypotenuse and the six trigonometric ratios?




How To Work With 30 60 90 Degree Triangles Education Is Around
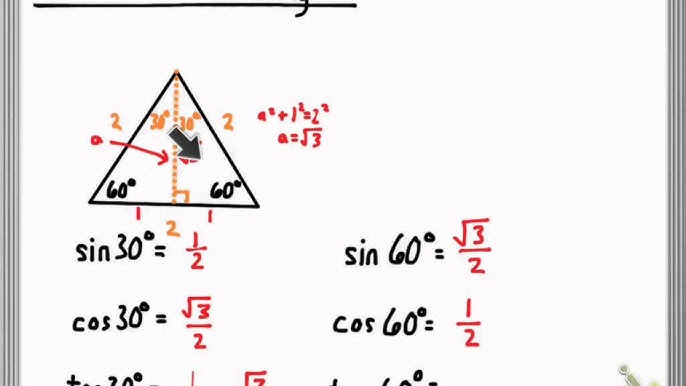
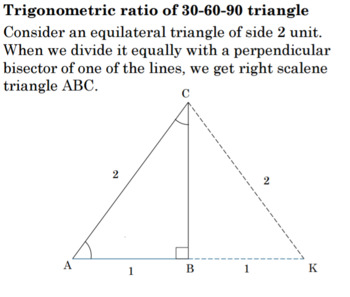
Answer (1 of 2) Refer to the above diagram Consider an equilateral triangle with length of each side equal to 2a An altitude cuts the equilateral triangle into two triangles In each triangle, the length of the shorter leg is a, while the length of the hypotenuse is 2a30 60 90 triangle ratio In 30 60 90 triangle the ratios are 1 2 3 for angles (30° 60° 90°) 1 √3 2 for sides (a a√3 2a) The trigonometric ratios for the angles 30°, 45° and 60° can be calculated using two special triangles An equilateral triangle with side lengths of 2 cm can be used to find exact values forThis trigonometry video tutorial provides a basic introduction into triangles It explains how to evaluate trigonometric functions such as sine and
Exact trigonometric ratios for 0°, 30°, 45°, 60° and 90° The trigonometric ratios for the angles 30°, 45° and 60° can be calculated using two special triangles An equilateral triangleTo learn more about Triangles enrol in our full course now https//bitly/Triangles_DMIn this video, we will learn 000 triangle017 proof of 306Using what we know about triangles to solve what at first seems to be a challenging problem Created by Sal Khan Special right triangles Special right triangles proof (part 1) Special right triangles proof (part 2) Practice Special right triangles triangle example problem This is the currently selected item
Exact trigonometric ratios for 0°, 30°, 45°, 60° and 90° The trigonometric ratios for the angles 30°, 45° and 60° can be found using two special triangles An equilateral triangle with All of these questions were solved using trigonometric functions however, I think there is a way to solve this using elementary geometry without trigonometric functions I tried to go somewhere with splitting $∠B$ into $$ triangles or a $0$ triangle but to no avail as it did not help me at allTrigonometric ratios table helps to find the values of trigonometric standard angles such as 0°, 30°, 45°, 60° and 90° It consists of trigonometric ratios – sine, cosine, tangent, cosecant, secant, cotangent These ratios can be written in short as sin, cos, tan, cosec, sec and cot



1




Fill In The Blanks In The 30 60 90 Triangle Below Side S Has A Length Of And Side Q Has Brainly Com
Learn about the trigonometric ratios of some specific angles including 0°, 30°, 45°, 60°, 90° Know the sin, cos, tan and other ratios for these angles with solved examples Trigonometric Ratios In Right Triangles Answer / Triangles Special Right Triangle Trigonometry If your calculator doesn't seem to be giving you the right answer, read your manual or ask someone for help And these trigonometric ratios allow us to find missing sides of a right triangle, as well as missing anglesIn Trigonometry, For all the trigonometric ratios we will find their values for some specific angles 0°, 30°, 45°, 60°, 90° Trigonometric ratios of angle 0° Let ABC is a rightangled triangle in which ∠B is the right angle and ∠C is an acute angle ϴ If the angle ϴ becomes 0° then line segment AC(Hypotenuse) will coincide with line segment BC(Base) and line segment AB




30 60 90 45 45 90 Special Right Triangles Free Printable Math Worksheets Teaching Geometry Printable Math Worksheets




The Complete Guide To The 30 60 90 Triangle
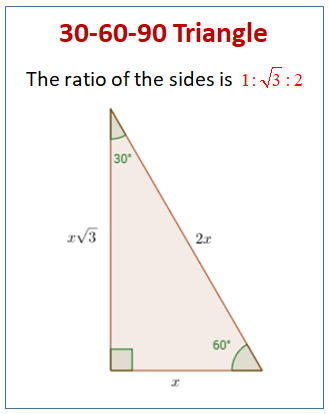
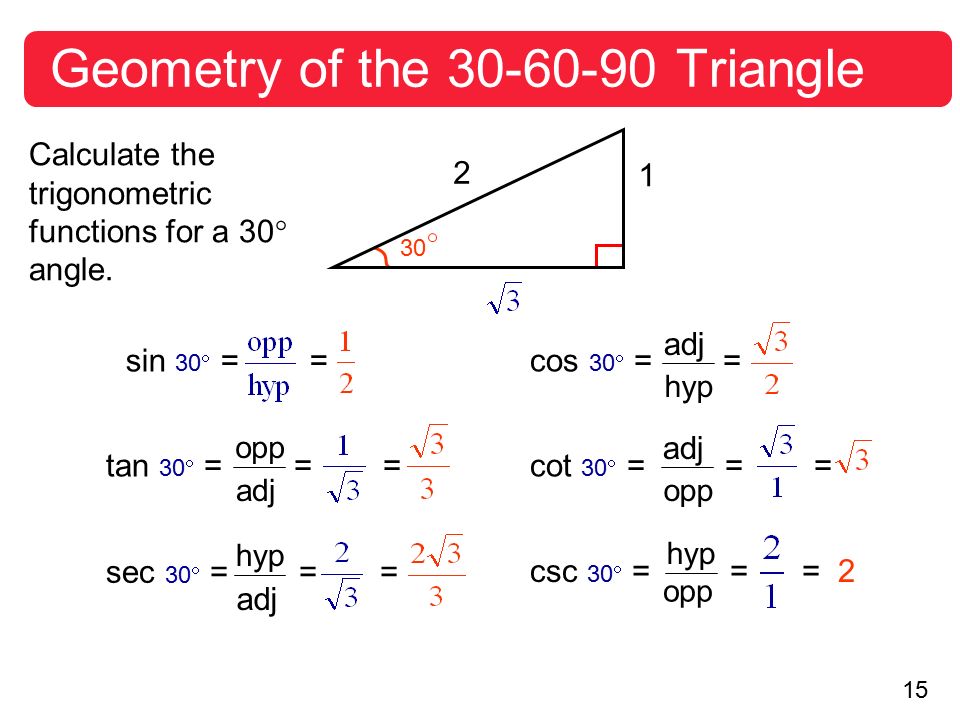
30 60 90 triangle rules and properties The most important rule to remember is that this special right triangle has one right angle and its sides are in an easytoremember consistent relationship with one another the ratio is a a√3 2a The trigonometric ratios for some particular angles such as 0°, 30°, 45°, 60° and 90° are presented below, which are generally used in mathematical computations Function 0°9 5 Trigonometric Ratios Geometry Objectivesassignment Find The Trigonometric Ratios Of Some Specific Angles Special Right Triangles Fully Explained W 19 Examples Trig Values For Paper 1 Triangle Method Gcse 30 60 90 Triangles Special Right Triangle Trigonometry Youtube 30 60 90 Triangles Special Right Triangle Trigonometry Youtube




Special Right Triangle 30 60 90 Mathbitsnotebook Geo Ccss Math




Special Right Triangles Fully Explained W 19 Examples
Trig ratios of special triangles Learn to find the sine, cosine, and tangent of triangles and also triangles Google Classroom Facebook Twitter30 60 90 Triangle The hypotenuse is 2 times as long as the short leg The ratio of the lengths of two sides in a right triangle Three common trigonometric ratios are sine, cosine, and tangent Tangent Ratio Let 'ABC, be a right triangle with acute angle A, thenExplain 3 If you are given a right triangle with an acute angle †, what two trigonometric




The Complete Guide To The 30 60 90 Triangle




How To Use The Special Right Triangle 30 60 90 Studypug
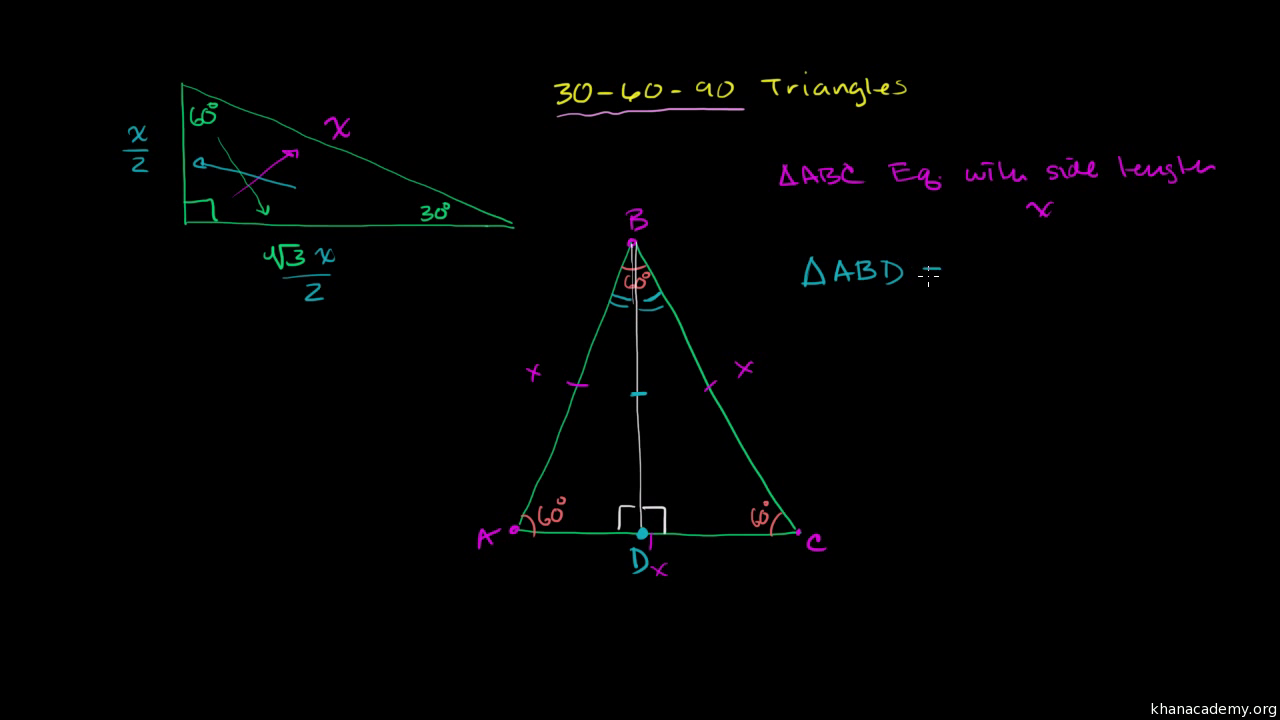
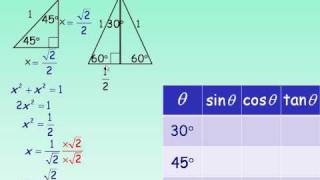
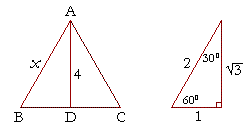
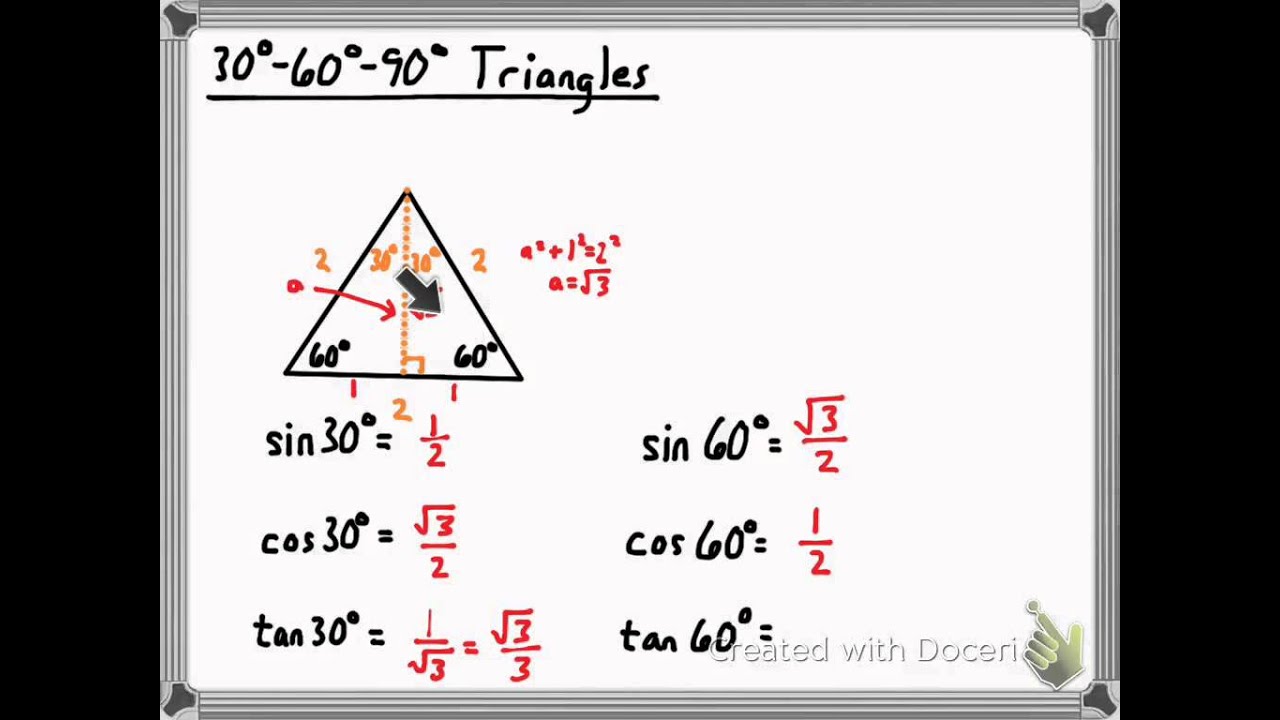
It is based on the fact that a 30°60°90° triangle is half of an equilateral triangle Draw the straight line AD bisecting the angle at A into two 30° angles Then AD is the perpendicular bisector of BC (Theorem 2) Triangle ABD therefore is a 30°60°90° triangle How do you find the sides of a Triangle?30° 60° 90° Triangle Theorem In a 30 ° 60° 90° triangle, the hypotenuse is twice as long as the shorter leg, and the longer leg is √3 times as long as the shorter leg It has been illustrated in the diagram shown below Trigonometric Ratios of Some Specific Angles Ratios for 0°, 30°, 45°, 60°, 90° Trigonometric Ratios of Some Specific Angles The term "trigonometry" comes from the Greek words "trigonon" (meaning "triangle") and "metron" (meaning "to measure") It is an area of mathematics that studies the relationship between a




Solved 2 Find The Unknown Side Lengths Of The Following 30 Chegg Com



13 3 2 The 30 60 90 Triangle Graphing Calculator By Mathlab User Manual
As trigonometry have different angles, for those different angles trigonometric ratios also have different values In the trigonometry the angles 0°, 30°, 45°, 60°, 90°, 1°, 135°, 150°, 180°, 210°, 225°, 240°, 270°, 300°, 315°, 330°, 360° are taken as standard angles We can find the trigonometric ratios of 0° and 90°How to derive and memorize the trigonometric ratios of the special angles, how to use the trig ratios of the special angles to find exact values of expressions involving sine, cosine and tangent values of 0, 30, 45, 60 and 90 degrees, How to find sin, cos, tan, cot, csc, and sec of the special angles, and multiples of 90, How to remember special angles, Grade 9 math, with video lessonsTriangle in trigonometry In the study of trigonometry, the triangle is considered a special triangleKnowing the ratio of the sides of a triangle allows us to find the exact values of the three trigonometric functions sine, cosine, and tangent for the angles 30° and 60° For example, sin(30°), read as the sine of 30 degrees, is the ratio of the side
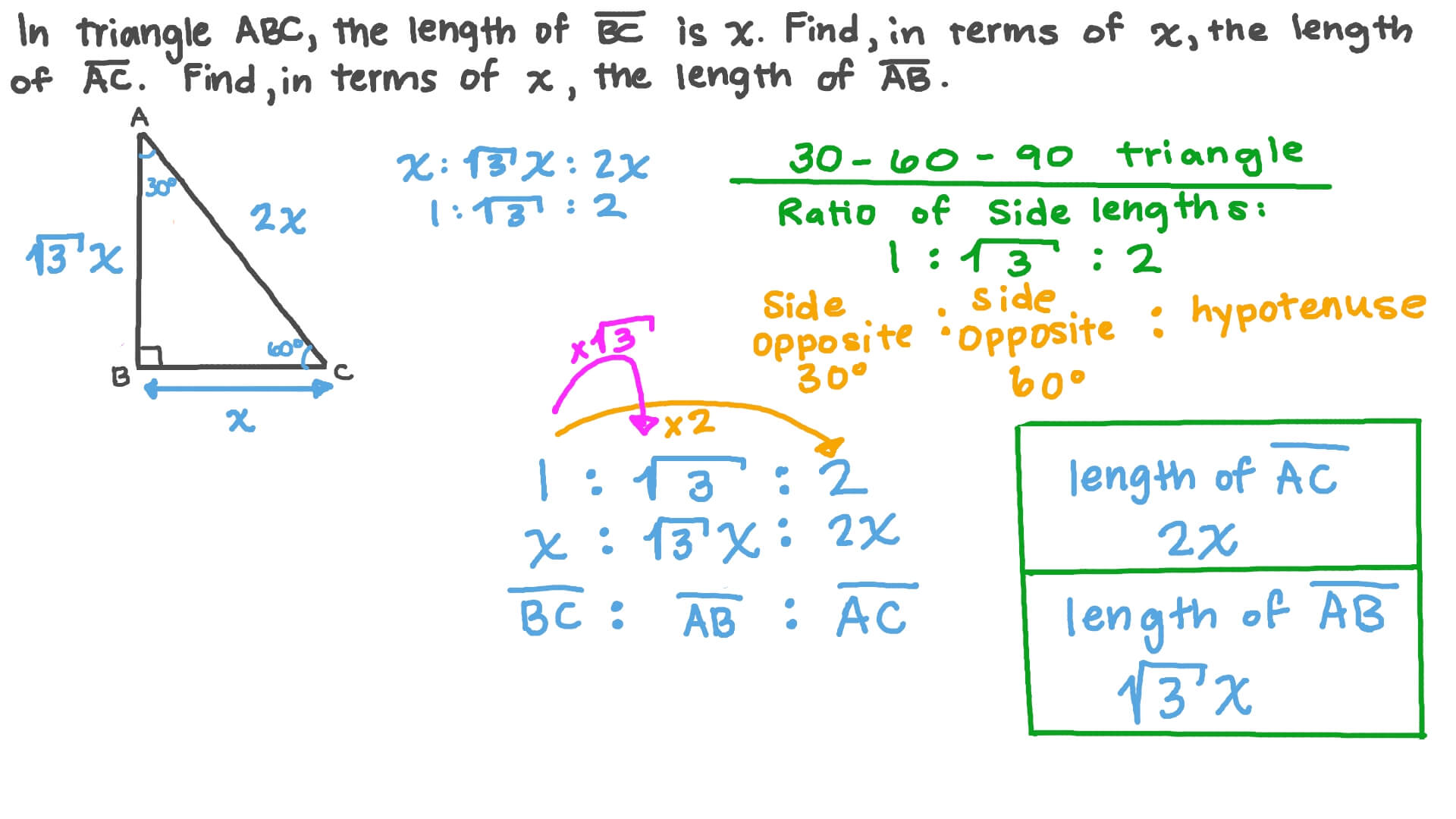



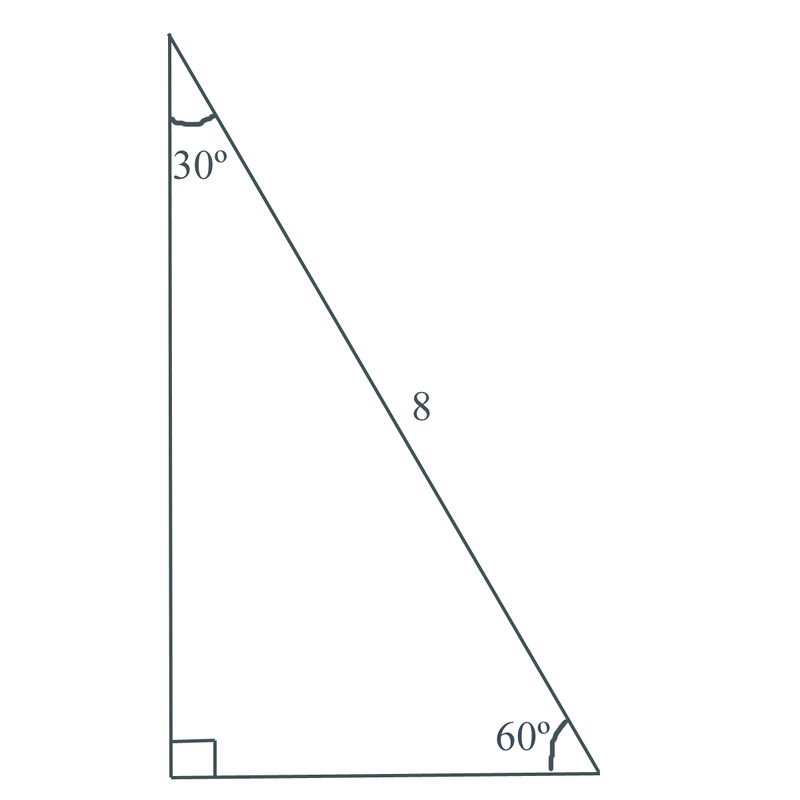
Question Video The Side Lengths Of 30 60 90 Triangles Nagwa




30 60 90 Triangle Explanation Examples
Trigonometric ratios of some specific angle are defined as the ratio of the sides of a rightangle triangle with respect to any of its acute angles Trigonometric ratios of some specific angle include 0°, 30°, 45°, 60° and 90° Now, let us learn how to find the trigonometric ratios Trigonometry is all about triangles or to more precise about the relation between the angles and sides of a rightangled triangle In this article we will be discussing about the ratio of sides of a rightangled triangle respect to its acute angle called trigonometric ratios of the angle and find the trigonometric ratios of specific angles 0°, 30°, 45°, 60°, and 90°(note the "sides" should instead be called "legs", since "sides" is ambiguous All portions of a triangle, the hypoten



30 60 90 Special Right Triangles Videos Worksheets Examples Solutions




30 60 90 Right Triangles Solutions Examples Videos
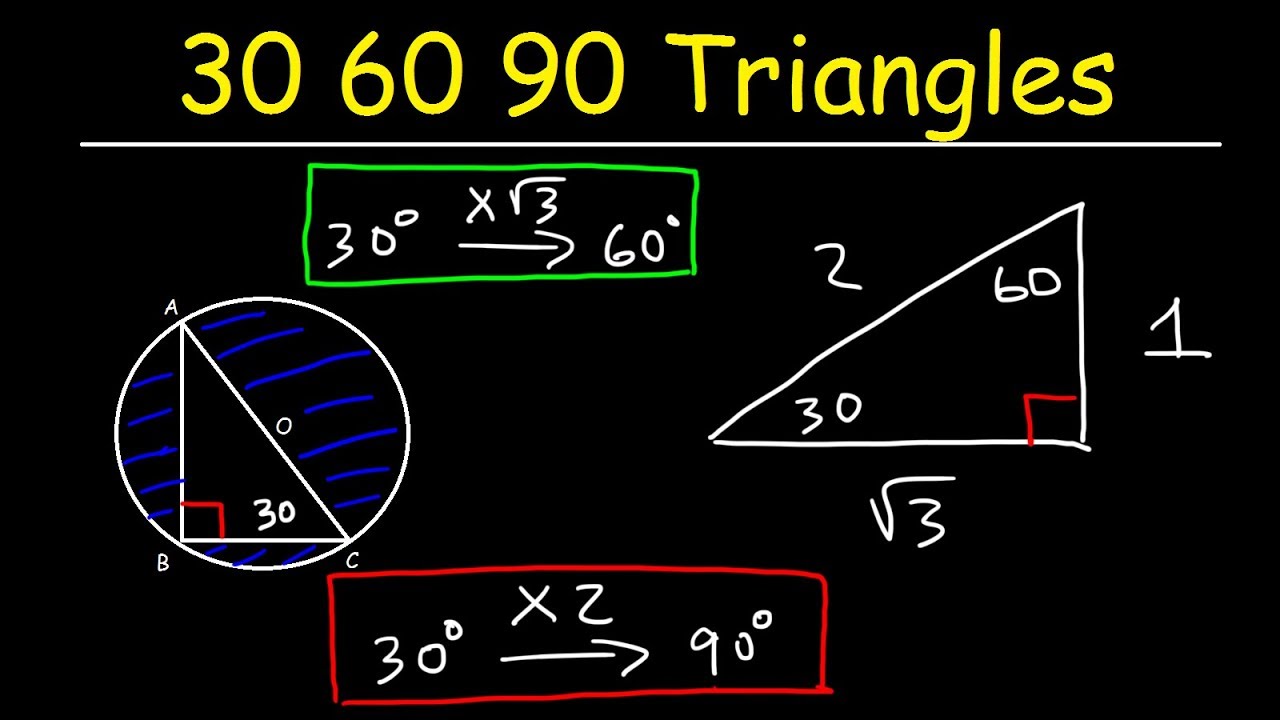
Now, by construction, each half of this triangle is a triangle Q What observations can you make about the relationship between the trigonometric ratios of 30 degrees and 60 degrees?Triangle Ratio A degree triangle is a special right triangle, so it's side lengths are always consistent with each other The ratio of the sides follow the triangle ratioThe other one is the 45 45 90 triangle These triangles are special triangles because the ratio of their sides are known to us so we can make use of this information to help us in right triangle trigonometry problems In the case of the triangle, their side's ratios are 1




How To Work With 30 60 90 And 45 45 90 Degree Triangles Dummies



Trig Ratios For 30 60 90 Triangles Youtube
This video screencast was created with Doceri on an iPad Doceri is free in the iTunes app store Learn more at http//wwwdocericomTrigonometric Ratios 30 60 90 Triangle In the case of the triangle, their side's ratios are 1 2 3 \sqrt3 3 We'll prove that this is true first so that you can more easily remember the triangle's properties A triangle is actually half of an equilateral triangle Triangle ABC shown here is an equilateral triangle The trigonometric ratios for 30^o, 45^o, and 60^o are based on some standard triangles sin, cos, and tan (and their reciprocals) are the ratios of the sides of these triangles Both 30^o and 60^o are based on an equilateral triangle with sides of length 2 and with one of the angles bisected The 45^o angle is based on an isosceles triangle with the equal sides having a



Trigonometric Ratios Of Special Angles 0 30 45 60 90 Video Lessons Examples And Solutions



Precalculus Notes Trig 3
Khan Academy Trigonometric Ratios In Right Triangles Answers 30 60 90 triangle equation ONETTECHNOLOGIESINDIACOM What is the tangent ratio? If one of the acute angles is 30 ̊, the other must be 60 ̊ When the side opposite the 30 ̊ angle is 1 unit, then the side opposite the 60 ̊ angle is units and the hypotenuse is 2 units 1 2 Nasir Islamic Mathematician 14 First, we will write the trigonometric ratios of the angle that measures 30 ̊A few more examples and an introduction to trianglesWatch the next lesson https//wwwkhanacademyorg/math/geometry/right_triangles_topic




Special Right Triangles
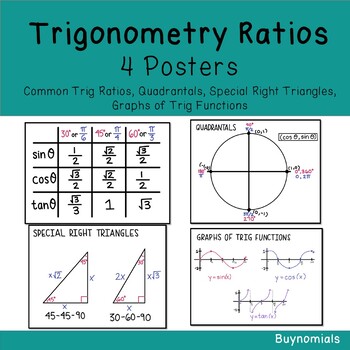



Trigonometric Ratios Quadrantals Special Right Triangles Graphs 4 Posters
Using what we know about triangles to solve what at first seems to be a challenging problemPractice this lesson yourself on KhanAcademyorg right no772 Chapter 13 Trigonometric Ratios and Functions 1 Explain what it means to solve a right triangle 2 Given a 30°60°90° triangle with only the measures of the angles labeled, can you find the lengths of any of the sides? Because it is a special triangle, it also has side length values which are always in a consistent relationship with one another The basic triangle ratio is Side opposite the 30° angle x Side opposite the 60° angle x * √ 3 Side opposite the 90° angle 2 x



Relationships Of Sides In 30 60 90 Right Triangles Ck 12 Foundation




A Quick Guide To The 30 60 90 Degree Triangle Dummies
Trigonometric Ratios 30 60 90 Triangle A right triangle (literally pronounced "thirty sixty ninety") is a special type of right triangle where the three angles measure 30 degrees, 60 degrees, and 90 degrees The triangle is significant because the sides exist in an easytoremember ratio 1 √3 3 2 That is to say, the hypotenuse is twice as long as the shorter leg, and the longer leg is theTrigonometric ratios in right triangles This means that in a right triangle having an acute angle of 28°, its opposite side is 469 thousandths of the hypotenuse, which is to say, a little less than




30 60 90 Triangle Theorem Properties Formula Video Lesson Transcript Study Com



30 60 90 Triangle Rules




The 30 60 90 Triangle Topics In Trigonometry
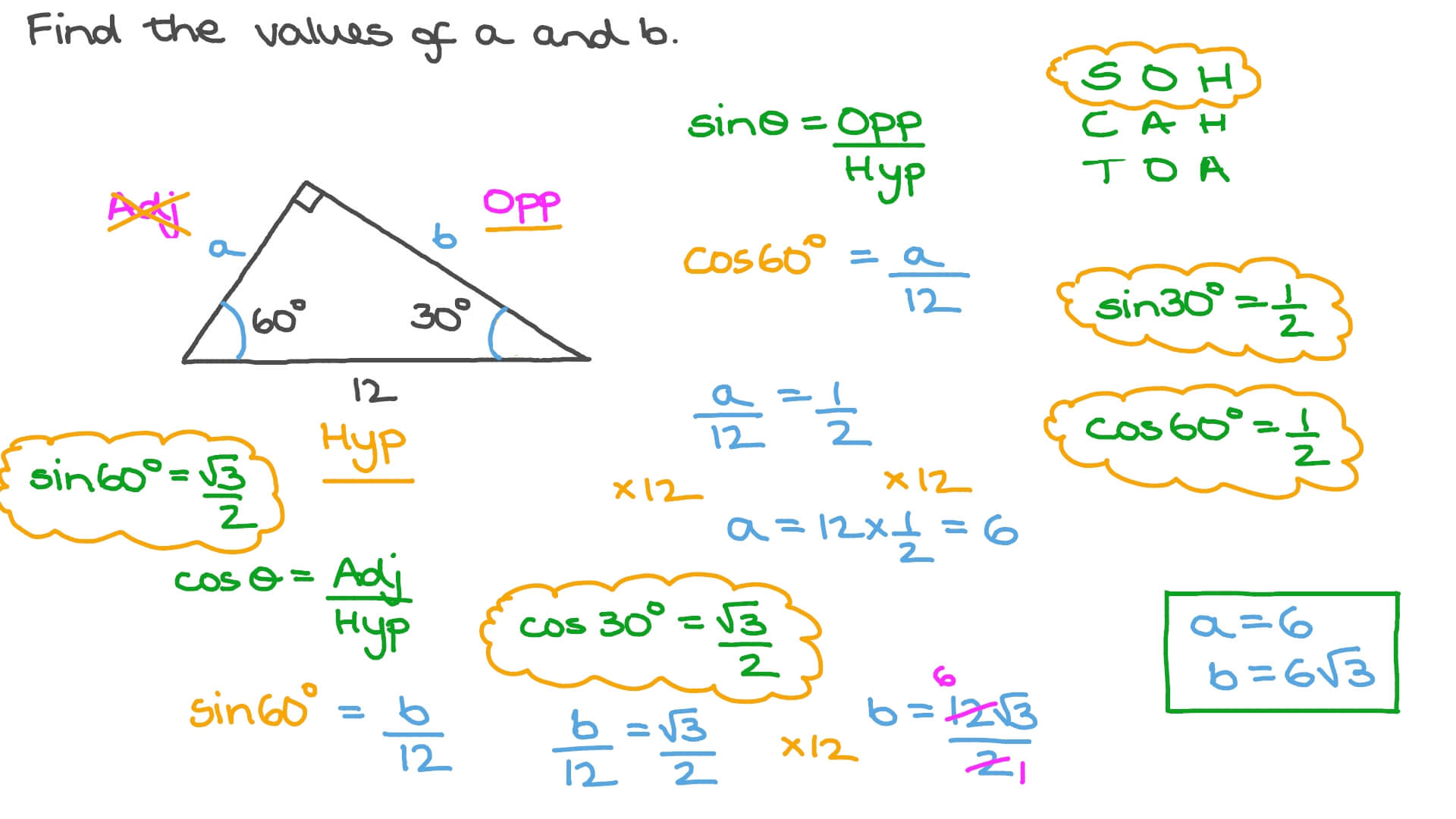



Question Video The Side Lengths Of 30 60 90 Triangles Nagwa




30 60 90 And 45 45 90 Triangles Youtube




Solved The Table Below Shows Trigonometric Relationships In Chegg Com




How Do You Find Trigonometric Ratios Of 30 45 And 60 Degrees Socratic



1
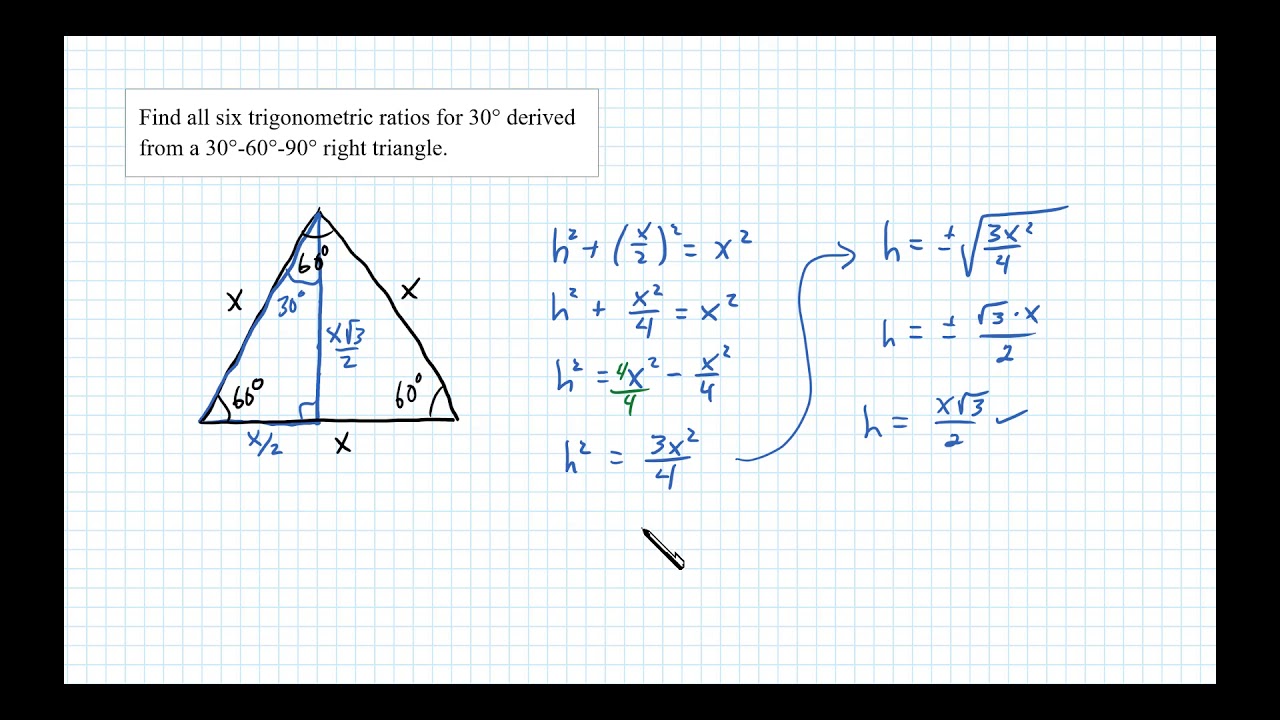



Trig Ratios For 30 Degrees Youtube




30 60 90 Triangle Theorem Ratio Formula Video




Trig Ratios Of Special Triangles Article Khan Academy
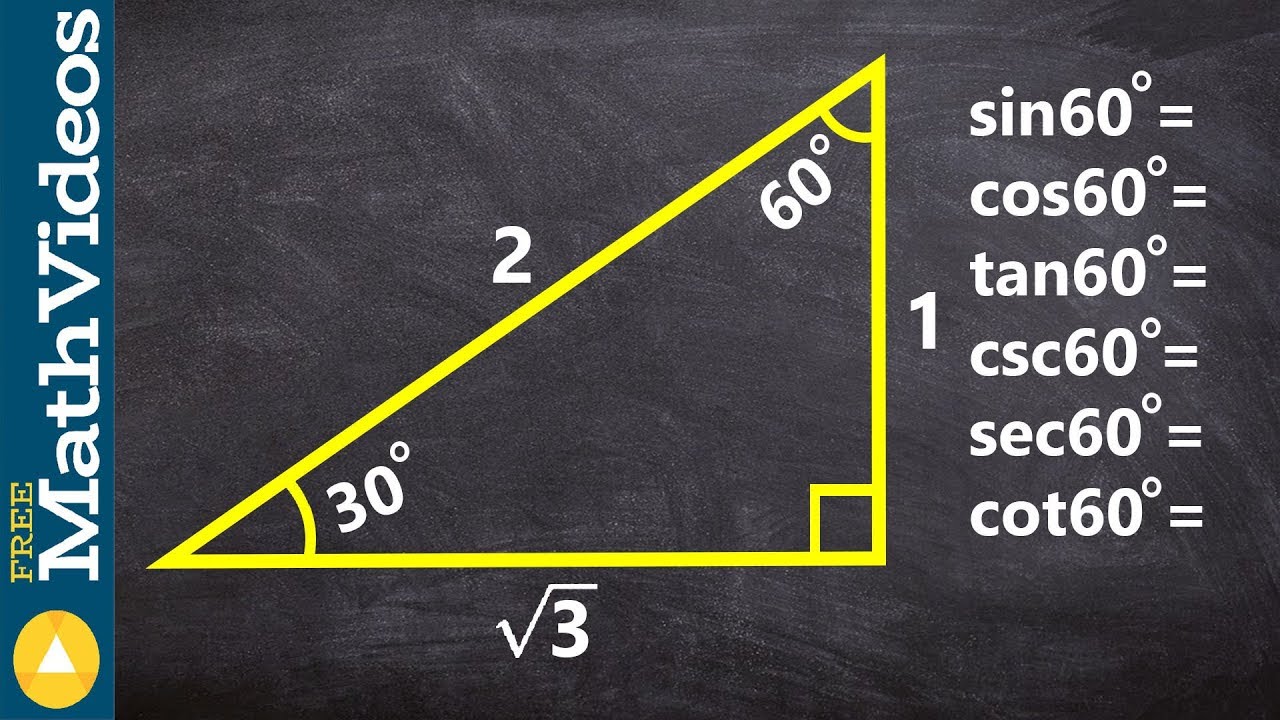



Learn How To Evaluate The Six Trig Functions Of A 30 60 90 Triangle Youtube




30 60 90 Triangle Definition Theorem Formula Examples




Trigonometric Ratios Of Special Angles 0 30 45 60 90 Solutions Examples Videos Worksheets Activi Trigonometric Functions Math Formulas Education Math




How To Work With 30 60 90 Degree Triangles Education Is Around




Special Triangles Used In Trigonometry
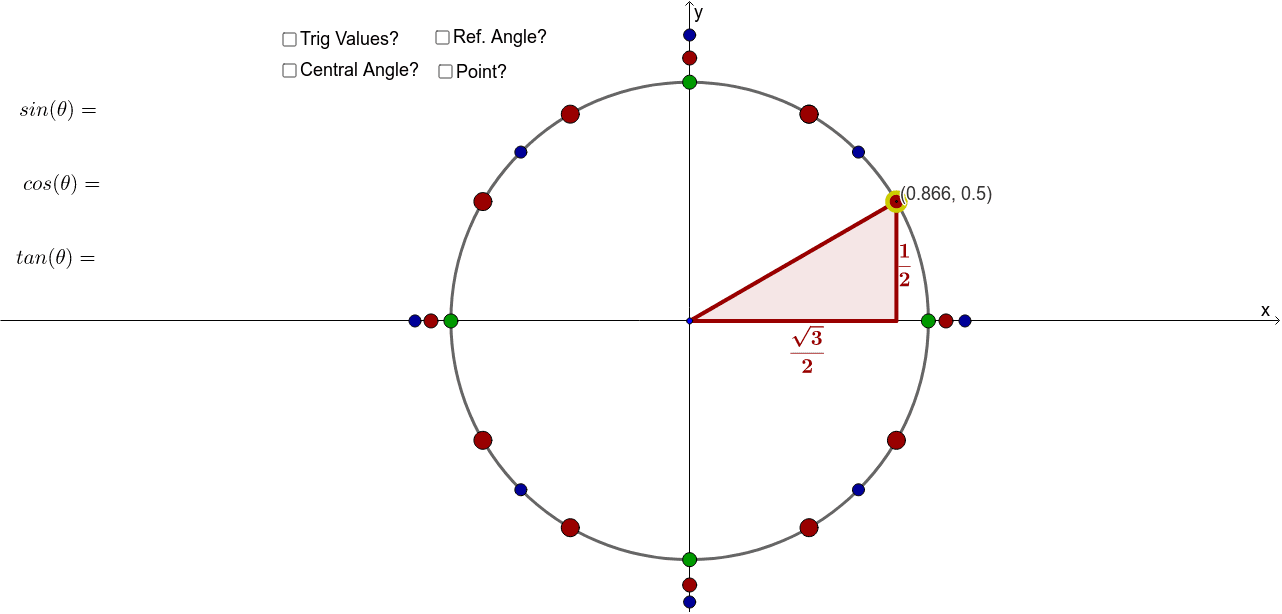



Explore The Unit Circle Geogebra
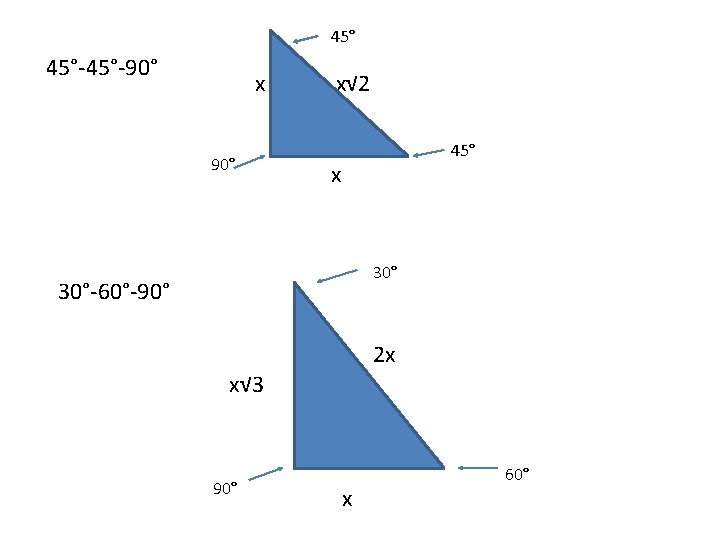



5 2 Trig Ratios In Right Triangles Objective



How Come In My Geometry Class I Was Taught Special Triangles 30 60 90 Triangles Had The Sides 1 1 2 3 2 But In My Trigonometry Class I Was Taught That The Sides For
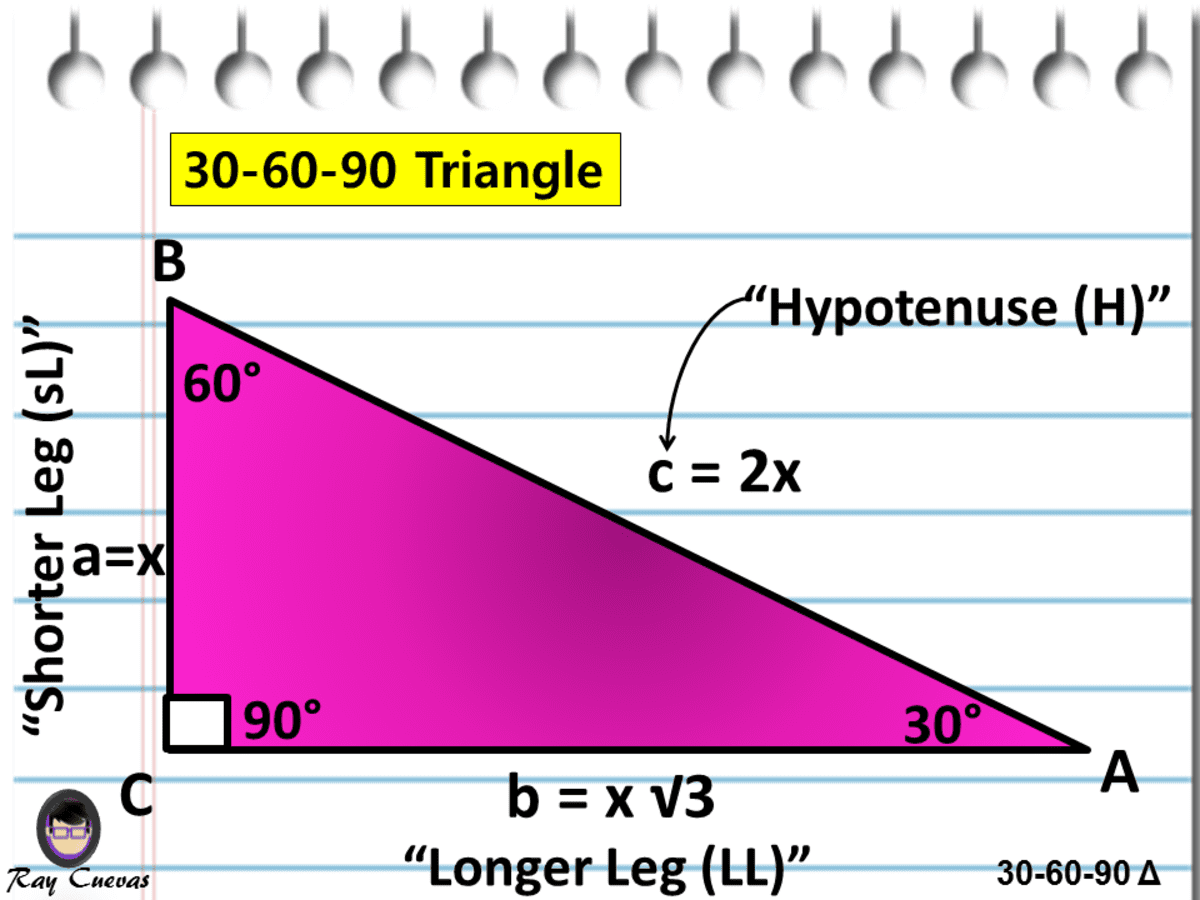



A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation
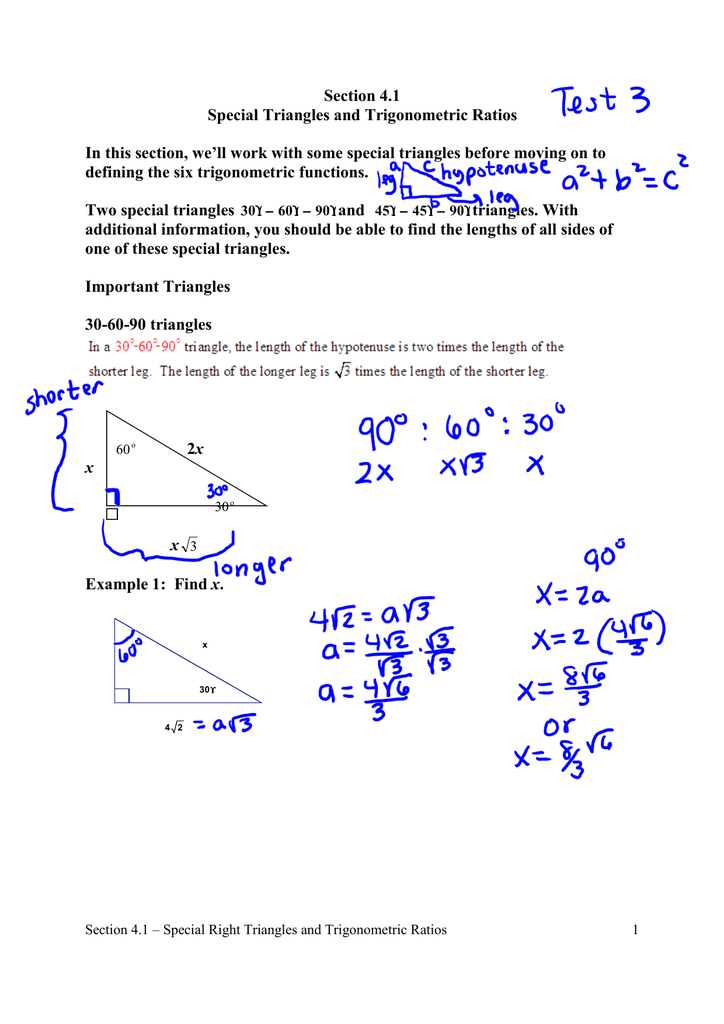



Section 4 1 Special Triangles And Trigonometric Ratios In This




Special Right Triangles Interactive Notebook Page Math Methods Teaching Geometry Teaching Math
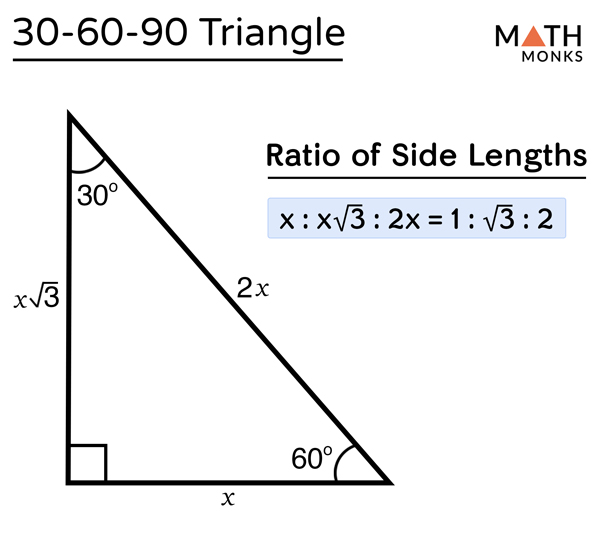



30 60 90 Triangle Definition Formulas Examples




30 60 90 Triangle Example Problem Video Khan Academy



The Easy Guide To The 30 60 90 Triangle



Precalculus Notes Trig 3



Precalc Week 17 Jonas Blog




30 60 90 Triangle Theorem Ratio Formula Video
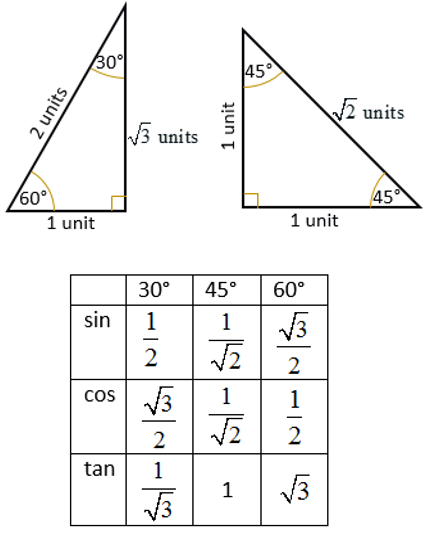



Trigonometric Ratios Of Special Angles 0 30 45 60 90 Video Lessons Examples And Solutions
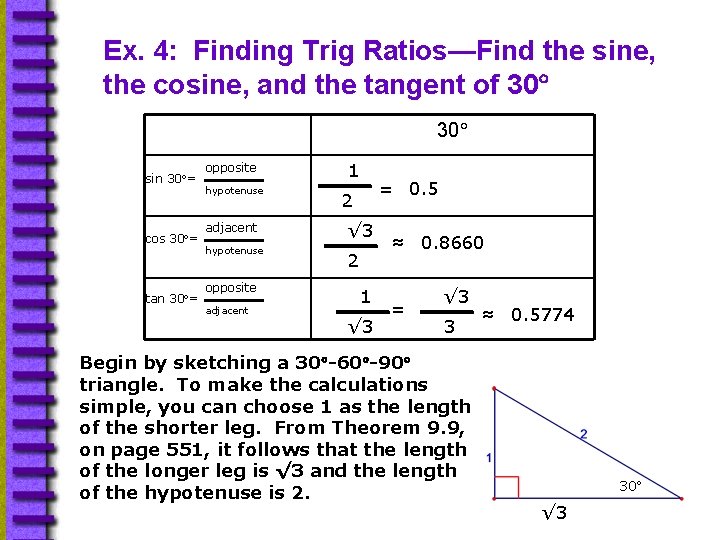



9 5 Trigonometric Ratios Geometry Objectivesassignment Find The



3




Right Triangle Trigonometry Ck 12 Foundation



The Easy Guide To The 30 60 90 Triangle



The 30 60 90 Triangle Topics In Trigonometry
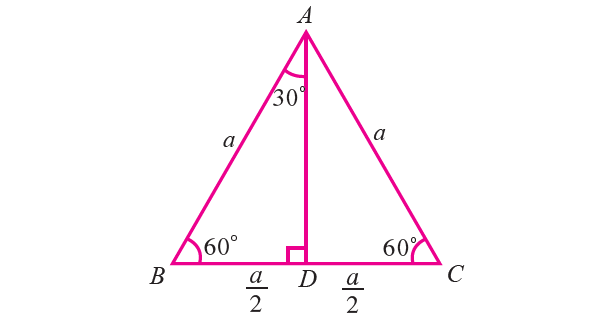



Trigonometric Ratios Of Some Specific Angles




30 60 90 Triangles Special Right Triangle Trigonometry Youtube




30 60 90 Triangles Spock S Guide To Math 4




30 60 90 Triangle Calculator Formula Rules
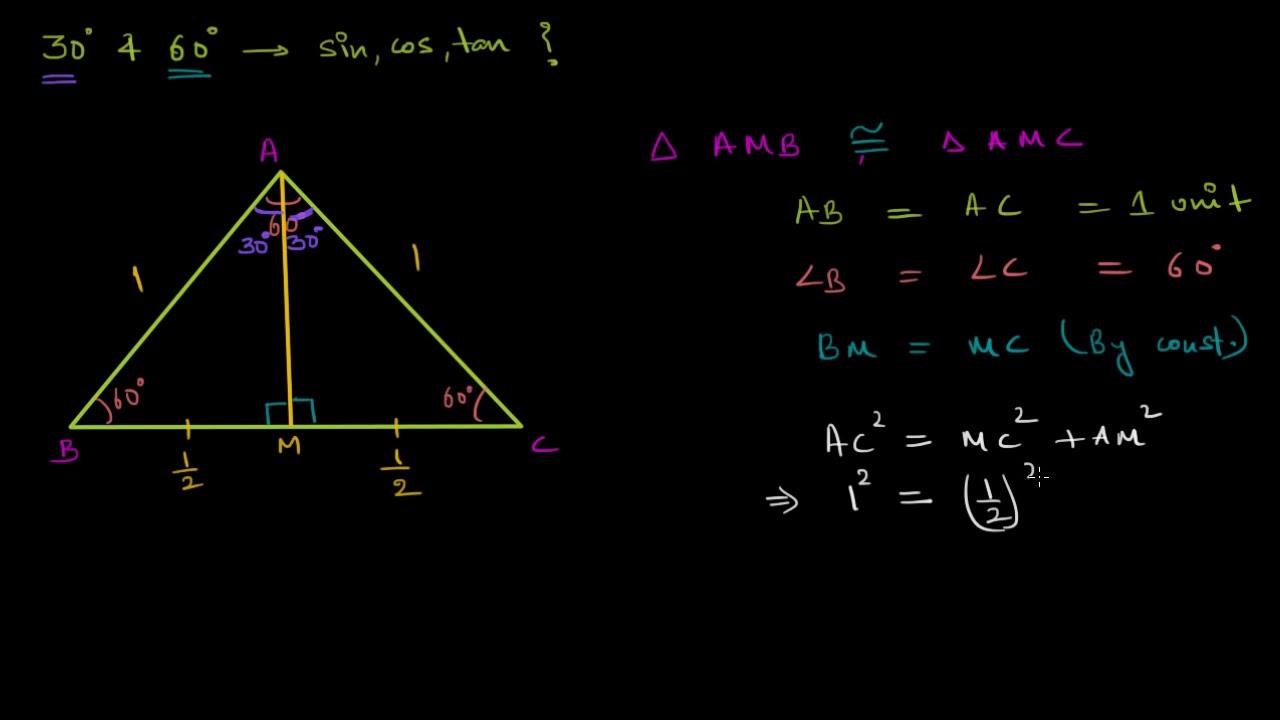



Trigonometric Ratios Of 30 And 60 Degrees Hindi Youtube



Right Triangle Trigonometry
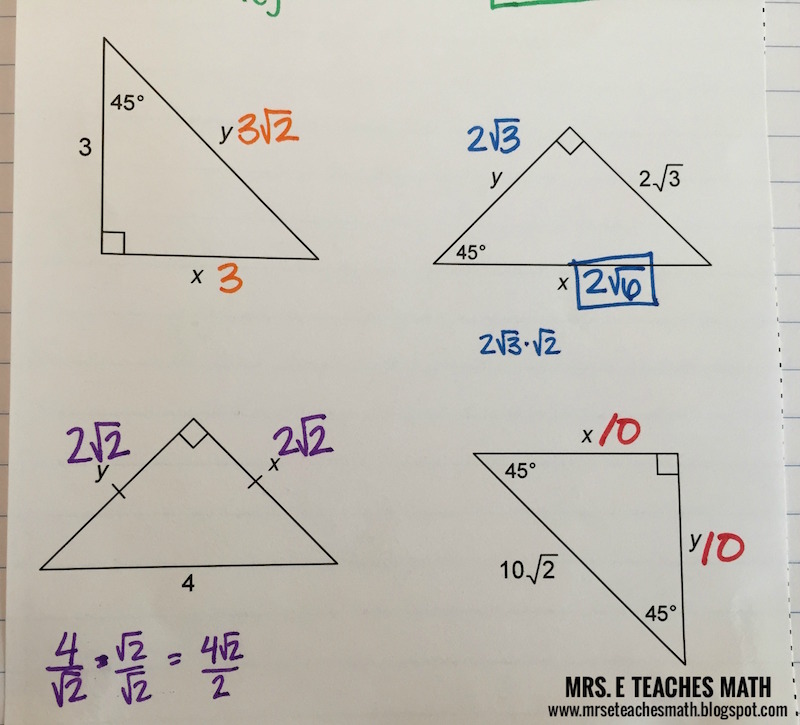



Right Triangles Interactive Notebook Pages Mrs E Teaches Math
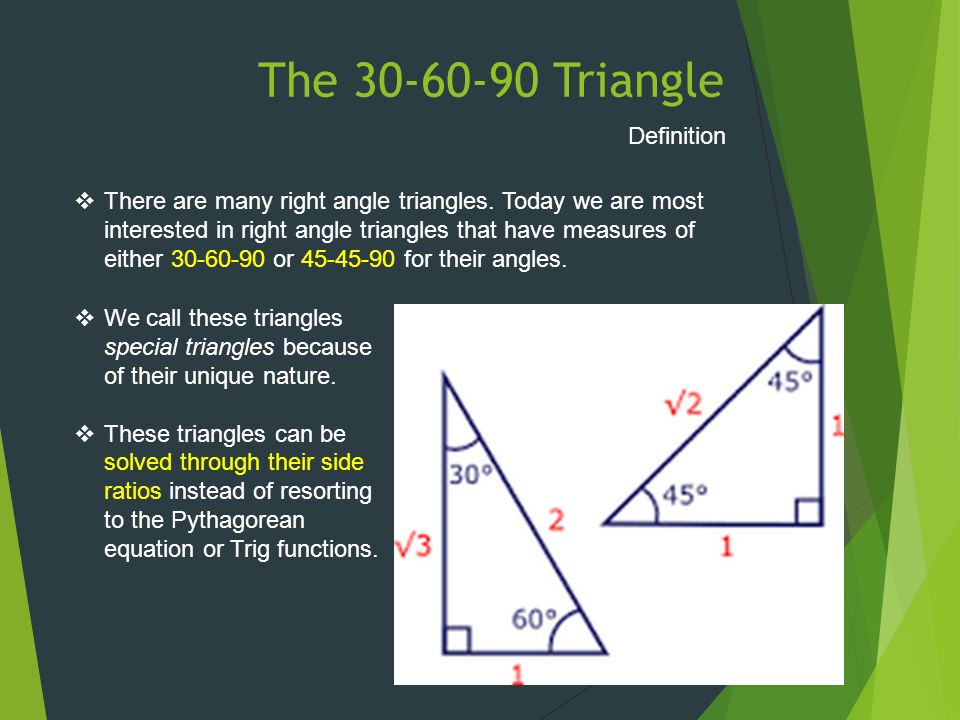



Special Right Triangles Definition And Use The Triangle Definition There Are Many Right Angle Triangles Today We Are Most Interested In Right Ppt Download



A 30 60 90 Triangle




Use The Theorems Of 30 60 90 Right Triangle To Solve The Unknown Variables X And Y And Find The Six Trigonometric Ratios 1 30csc30º
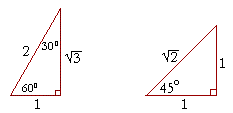



The 30 60 90 Triangle Topics In Trigonometry



45 45 90 And 30 60 90 Triangles Zona Land Education
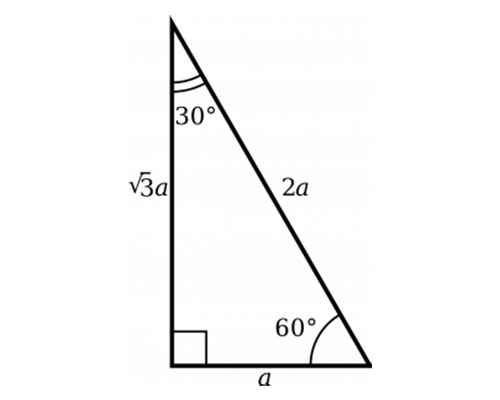



30 60 90 Triangle Sides Examples Angles Full Lesson




30 60 90 Triangle Formulas Rules And Sides Science Trends
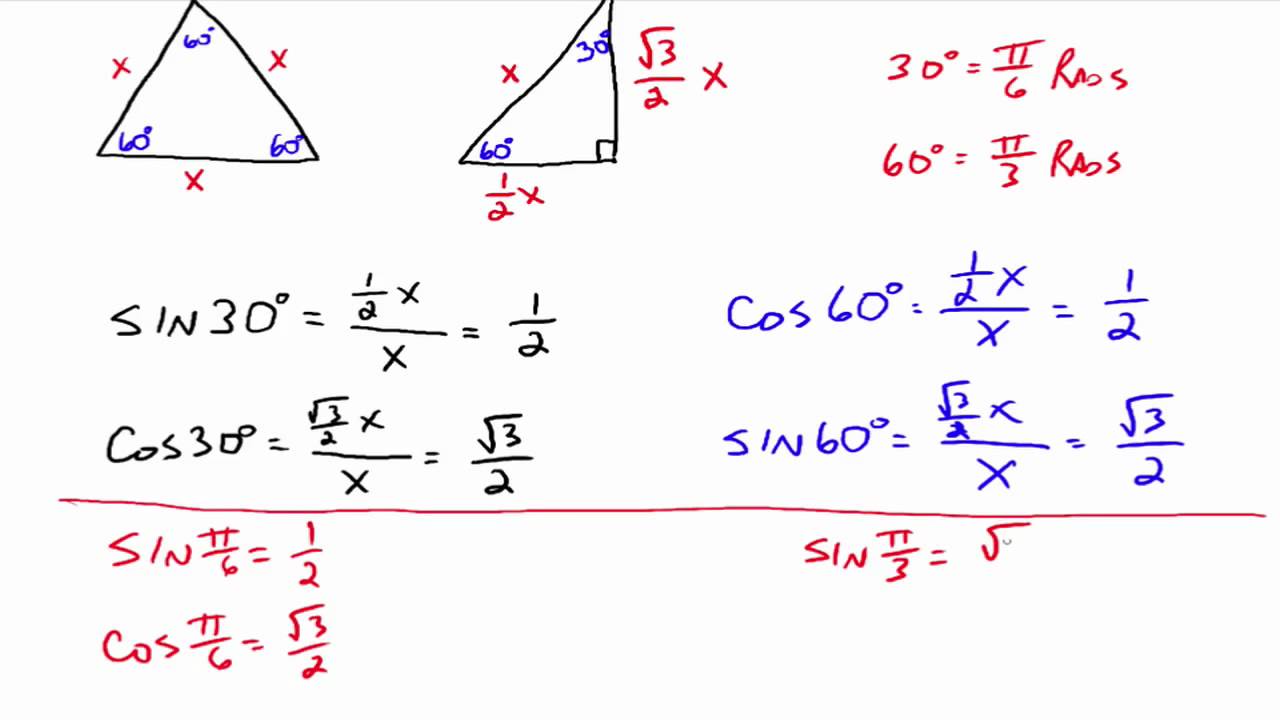



Trigonometric Ratios Of Special Angles 0 30 45 60 90 Video Lessons Examples And Solutions




30 60 90 Triangles




Trig Values For Paper 1 Triangle Method Gcse




Exact Trigonometry Ratios Part 1 0 30 45 60 And 90 Degrees Youtube



The Easy Guide To The 30 60 90 Triangle



30 60 90 Triangles Special Right Triangle Trigonometry Youtube




30 60 90 Triangle Theorem Ratio Formula Video



The 30 60 90 Triangle Topics In Trigonometry




30 60 90 Triangle Explanation Examples



13 3 2 The 30 60 90 Triangle Graphing Calculator By Mathlab User Manual




8 4 Special Right Triangles Inc Maine South Plane Geometry




Special Right Triangles



Right Triangle Trigonometry Evaluate Trigonometric Functions Of Acute Angles And Use A Calculator To Evaluate Trigonometric Functions Use Ppt Download




A Quick Guide To The 30 60 90 Degree Triangle Dummies
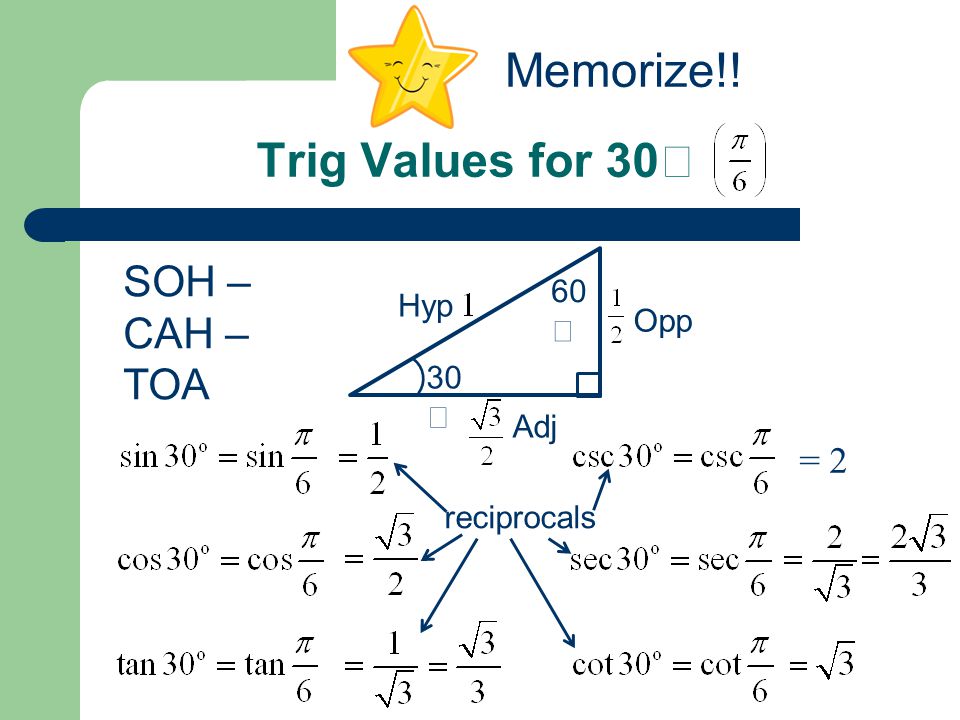



7 3 Special Angles 30 60 Equilateral Triangle 60 Angles W Sides 1 Drop Perpendicular Bisector To Form 1 60 60 Ppt Download




30 60 90 Triangle Explanation Examples




Special Angles




Special Triangles Used In Trigonometry
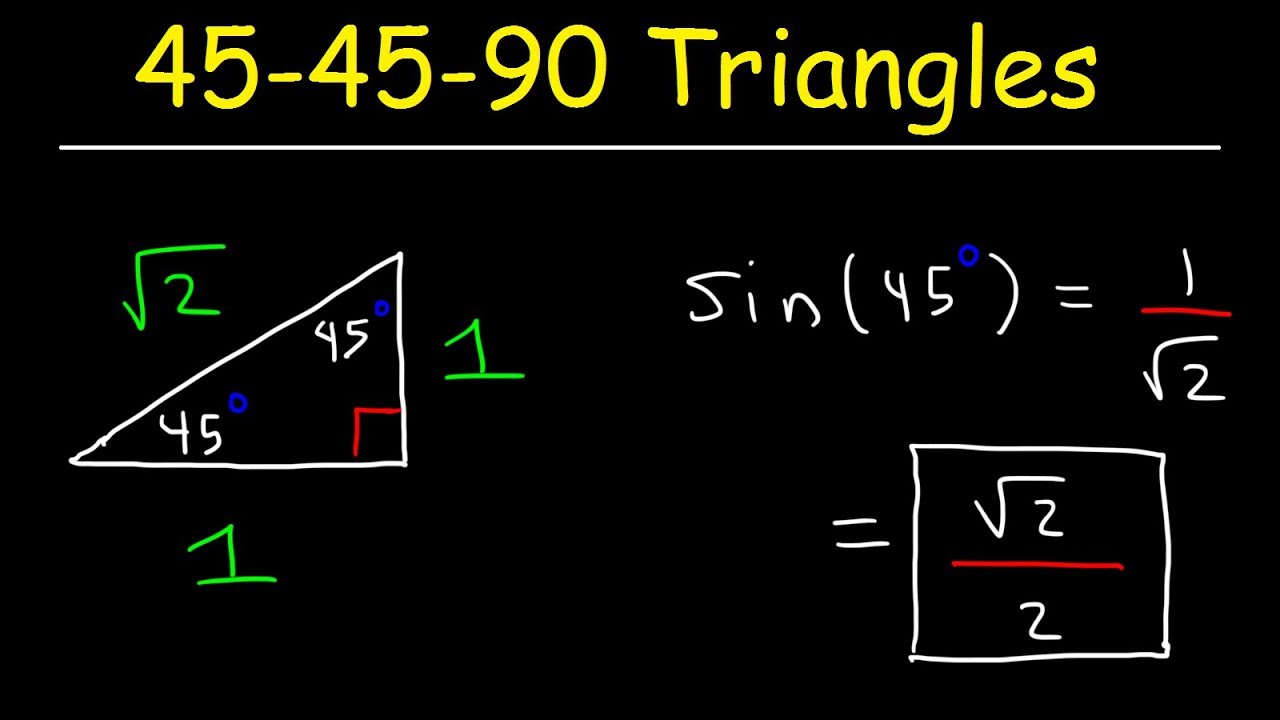



30 60 90 Triangles Special Right Triangle Trigonometry Youtube




Special Right Triangles Proof Part 1 Video Khan Academy




30 60 90 Triangle Definition Theorem Formula Examples
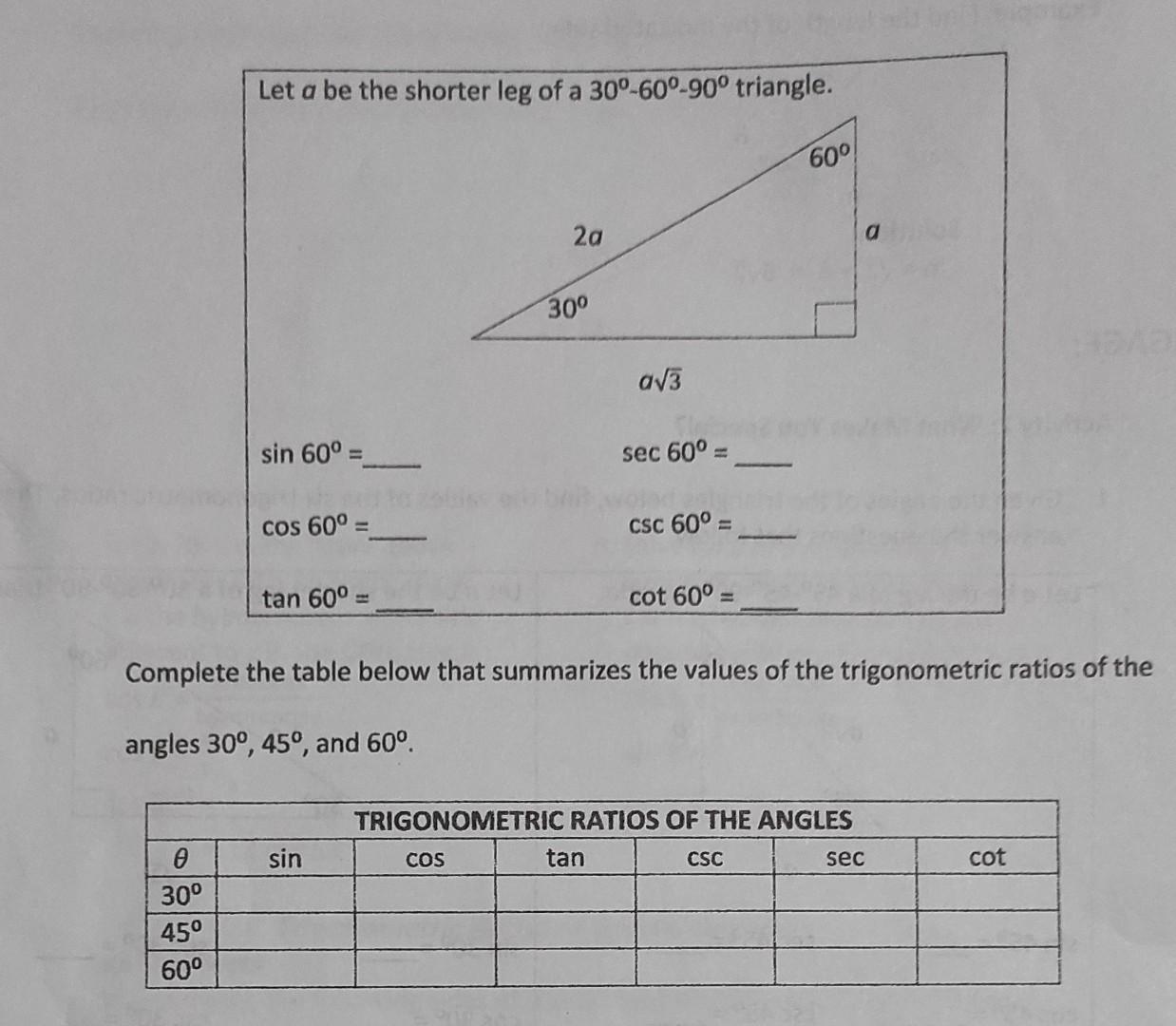



Solved Let A Be The Shorter Leg Of A 30 60 90 Triangle Chegg Com




30 60 90 Triangle Theorem Properties Formula Video Lesson Transcript Study Com
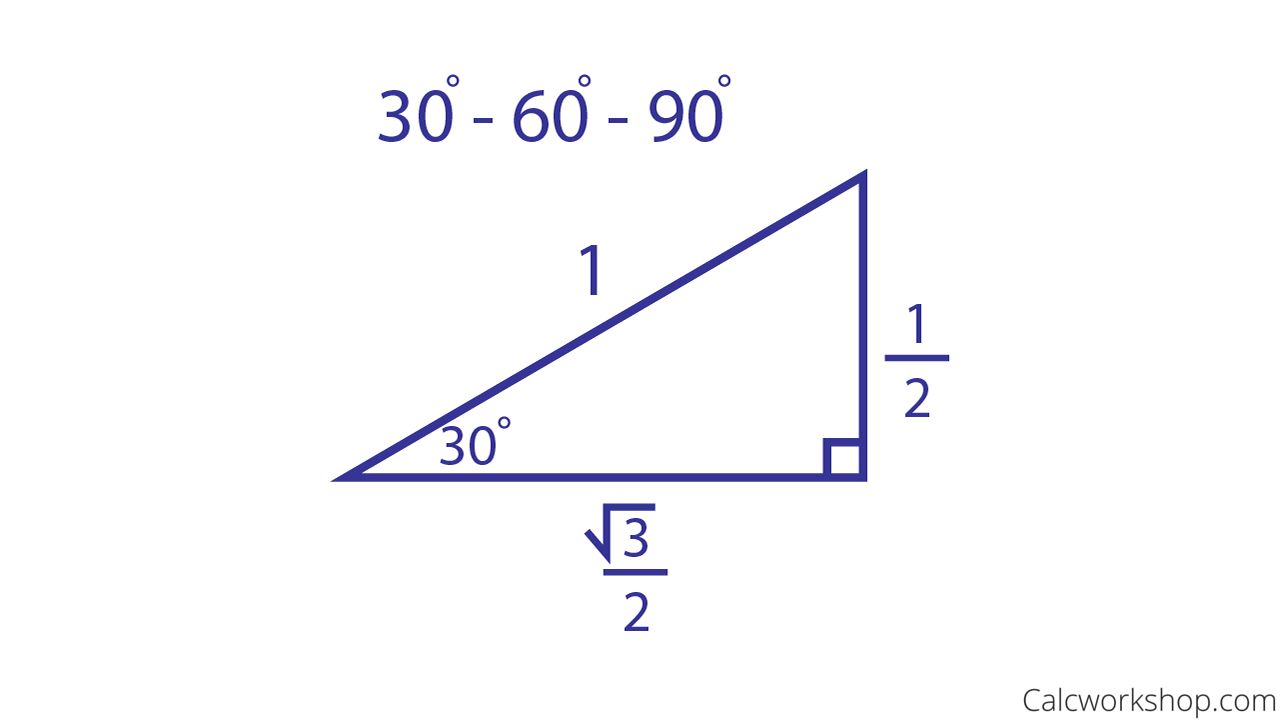



Unit Circle W Everything Charts Worksheets 35 Examples




30 60 90 Right Triangles Free Math Help



Chapter 3 Trigonometric Functions Of Angles Section 3 2 Trigonometry Of Right Triangles Ppt Download




30 60 90 Right Triangle Side Ratios Expii



Trigonometry Of 30 60 90 Right Triangle 1 Lesson Plan G Srt C 6 Tpt




30 60 90 Right Triangles Ck 12 Foundation



1



Trig Ratios For 30 60 90 Triangles Youtube




The 30 60 90 Triangle Topics In Trigonometry




30 60 90 Triangle Rules Sides Ratio Of A 30 60 90 Triangle Video Lesson Transcript Study Com




What Is Cos 60 How To Prove It Quora



0 件のコメント:
コメントを投稿